題292中正資管 The following are eight consecutive weeks earnings in dollars of three door-to door vacuum cleaner salepersons employed by a given firm: A: 2
題233清大科管 The sales volume In thousand dollars for three different types of in-store promotions is shown below. To test the effect of in-store promotio
92朝陽企管 How many times would you expect to roll a fair die before all 6 sides appreared at least once? 6面公平骰子都至少出現一次,投擲次數的期望值為何?
假設汽車到達停車場的車數事件滿足波氏公設 已知一小時平均有10部車到達,令X為半小時到達的車數,則X之機 率函數為何? 2上題1之任意二部車的間隔時間為隨機變數設定為Y,其機至函數為 何?5% Hi,好帳戶不能只有我知道!和我一起加入樂天,領取交友獎勵金200元及享有新戶優惠! 推薦序號: IVJ9
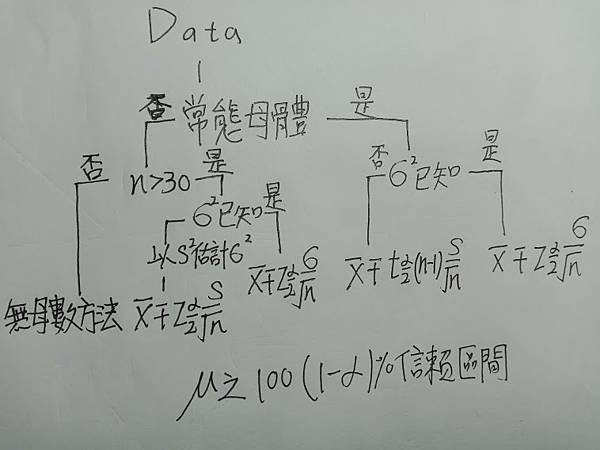
A 99% confidence interval estimate can be interpreted to mean that Alf all possible samples are taken and confidence interval estimates are developed,
淡江管科 自某一母體中抽取10個樣本資料如下:60,80,75,20,95,35,50,65,75,55試求此樣本資料之下列有關統計數值: 1平均數 2標準差 3第90個百分數值 答: 一銀ileo數位帳戶快拿100元被推薦禮新戶100元之前已有一銀非ileo帳戶舊戶50元,2021/12/31前掃
一銀ileo數位帳戶快拿100元被推薦禮新戶100元之前已有一銀非ileo帳戶舊戶50元,2021/12/31前掃碼線上開戶成功並下載ileo推薦碼LqGatx的app完成登入就有100元被推薦禮60天內入帳好康優惠!ileo開戶 被推薦禮:新戶100元,已有一銀帳戶但首次申請之用戶50元,新舊戶定
台北市某大廈的防盜警報響時,第一保全公司有下列二種假設決策: 一切安好,僅防盜設備線路受到干擾,所以不必派人處理, 有小偷潛入,立即通知警局,並派人處理, 試問上述何者為H0? 何者為H1? 為什麼? 輔大經研 答: 錯誤拒絕小偷潛入,會造成較大損失,應為虛無假設H0我們希望有充分證據才去推翻虛無假
